Logarithm – Definition, Rules , Furmulas and Examples

Last Updated : 17 Dec, 2024

Logarithm is a mathematical function that represents the exponent to which a fixed number, known as the base, must be raised to produce a given number. In other words, it is the inverse operation of exponentiation. Logarithm is a versatile tool that simplifies the calculations by converting complex multiplicative processes into more manageable additive ones.

Mathematical Expression for Log

If aⁿ = b then log or logarithm is defined as the log of b at base a is equal to n. It should be noted that in both cases base is ‘a’ but in the log, the base is with the result and not the power.

aⁿ = b ⇒ log_ab = n

a is Base

b is Argument

a and b Positive Real Numbers

n is Real Number

Table of Content

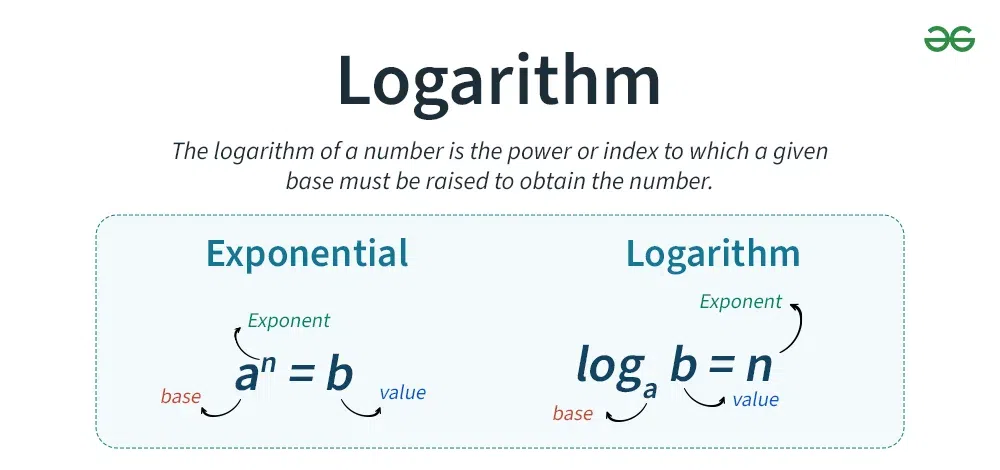
Articles related to Logarithms:

Conversion from Exponential to Log Form

If a number is expressed in the exponential form for example an = b where a is the base, n is the exponent and b is the result of the exponent then to convert it into the logarithmic form the base ‘a’ remains base in logarithm, the result ‘b’ becomes an argument and the exponent ‘n’ becomes the result here.

aⁿ = b ⇒ log_ab = n

Conversion from Log to Exponential Form

If the expression is in logarithmic form then we can convert it into exponential form by making the argument as a result and the result of logarithm becomes the exponent while the base remains the same. It can be better understood from the expression mentioned below:

log_ab = n ⇒ aⁿ = b

Types of Logarithm

Depending upon the base, there are two types of logarithm, which are,

Common Logarithm

The logarithm with base 10 is known as Common Logarithm. It is written as log₁₀X. The common logarithm is generally written as log only instead of log₁₀.

Let’s see some examples.

log10 = log₁₀10 = 1
log1 = log₁₀1 = 0
log1000 = log₁₀1000 = 3

Natural Logarithm

The logarithm with base e, where e is a mathematical constant is called Natural Logarithm. It is written as log_eX. The natural logarithm is also written in the abbreviated form as ln i.e. log_eX = ln X.

Let’s see some example:

log_e2 = X ⇒ e^X = 2
log_e5 = y ⇒ e^y = 5

Difference between Common Logarithm (Log ) and Natural Log (ln)

The basic difference between log and ln is tabulated below:

Log	Ln
Log is the logarithmic expression at base 10.	Ln is the logarithmic expression at base e.
It is given as log₁₀X	It is given as log_ex
It is called a common log and is represented as log x	It is called natural log and is represented as ln x
It is mostly used for solving large numbers and simplifying calculations.	It is less commonly used

Learn More: Difference Between Log and Ln

Rules of Logarithm | Log Rules

The common properties or rules of Log are :

Product Rule
Division Rule
Power Rule
Change of Base Rule
Base Switch Rule
Equality of Log
Number raised to Log Power
Negative Log Rule

Read in Detail: Logarithm Rules | List of all the Log Rules with Examples

Apart from the above-mentioned properties, there are some other properties of Log. Using these properties we can directly put their values in any equation. These properties are mentioned below:

Log 1 =0 This property of log states that the value of Log 1 is always zero, no matter what the base is. This is because any number raised to power zero is 1. Hence, Log 1 = 0.
Log_aa This Property of log states that if the base and augment of a logarithm are the same then the logarithm of that number is 1. This is because any number raised to power 1 results in the number itself. Hence, ln e = log_ee = 1 r.
The value of log of zero is not defined as there is no such number when raised to any power that results in zero. Hence, log 0 = Not defined.

Log and Antilog Table

Log Table

Log Table is used to find the value of the log without the use of a calculator. The log table provides the logarithmic value of a number at a particular base.

A log table has mainly three columns. The first column contains two-digit numbers from 10 to 99, the second column contains differences for digits 0 to 9 and hence called the difference column and the third column contains mean difference from 1 to 9 and hence called mean difference column.

The log table for base e is called the natural logarithm table and that for base 2 is called the binary log table.

The logarithmic value of a number contains two parts named characteristics and mantissa both separated by a decimal. Characteristic is the integral part written o the left side of the table and can be positive or negative while the mantissa is the fraction or decimal that is always positive.

Read More : Log Table

Anti Log Table

Antilog is the process of finding the inverse of the log of the number. This is used when the number is already given in log value and we need to find out the number for which log value is given. If log a = b then a = antilog (b).

Antilog table is helpful in finding the Antilog value without using the calculator. The antilog table also consists of 3 columns among which the first column contains numbers from .00 to .99, the second block which is the difference column contains digits from 0 to 9, and the third block which is the mean difference column contains digits from 1 to 9.

Read More: Antilog Table

Logarithmic Function

A logarithmic function is the inverse of an exponential function and is defined for positive real numbers with a positive base (not equal to 1). The logarithmic function to the base b is represented as f(x) = log⁡_b(x), where x>0 and b>0. In this function, X is the argument of the logarithm, and b is the base.

Graph of Logarithmic Function

We know that the domain of Logarithmic Function is (0, ∞) and its range is a set of all real numbers. If we plot the graph using the set of domain and range we find that the graph of the logarithmic function is just the inverse of the graph obtained for the exponential function.

Logarithmic Graph

This indicates the inverse relationship between exponential and logarithmic functions. Also, the logarithmic graph is symmetric around the line y = x. We know that the value of log 1 is zero at any base value. Hence it has an intercept (1,0) on the x-axis and no intercept on the y-axis as log 0 is not defined.

Properties of Logarithmic function

There are the following properties of the logarithmic Graph of function log_ax

In logarithmic function base a > 0 and a ≠ 1
The graph of logarithmic function increases when a > 1 and decreases in the range 0 < a < 1.
The domain of the function is a set of all positive numbers greater than zero.
The range of the curve is a set of all real numbers.

Articles related to Logarithms:

Laws of Logarithm

Product rule

Quotient Rule

Power Rule

Change of Base Rule

Solved Examples on Logarithms

Example 1: Find log_a16 + 1/2 log_a225 – 2log_a2
Solution:

log_a16 + 1/2 ✕ 2log_a15 – log_a2²

⇒ log_a16 + log_a15 – log_a4
⇒ log_a(16 ✕ 15) – log_a4

⇒ log_a(16 ✕ 15/4) = log_a60

Example 2: Solve log_b3 – log_b27
Solution:

log₂3 – log₂48

⇒ log₂(3/48)
⇒ log₂(1/16)
⇒ log₂(-16)

⇒ -log₂2⁴
⇒ -4log₂2 = -4

Example 3: Find x in log_bx + log_b(x – 3) = log_b10
Solution:

Given log_bx + log_b(x – 3) = log_b10

⇒ log_b(x)(x – 3) = log_b10
⇒ (x)(x – 3) = 10
⇒ x²– 3x – 10 = 0
⇒ x² – 5x + 2x – 10 = 0
⇒ x(x – 5) + 2(x – 5)

⇒ (x – 5)(x + 2) = 0
⇒ x = 5, -2

People Also Read,

Logarithm – FAQs

What are Logarithms?

Logarithm is the inverse of exponential which is used to find out to what power a base must be raised to yield a particular value.

What are Different Types of Logarithms?

There are two types of Logarithms:

Common Logarithm (Base 10)

Natural logarithm (Base e)

Who Invented Logarithm?

Logarithm was invented by Scottish Mathematician John Napier in 1614.

Can Logarithm be Negative?

No the argument of logarithm can’t be negative, however, log of any number can give a negative value.

What are Logarithms used for?

Logarithm is used to find the power a number must be raised to yield a particular result. It is useful in finding pH level, exponential growth or decay, etc.

What is the value of log_a0?

Logarithm does not take 0 and negative numbers as its input hence log_a0 is not defined.

Laws of Logarithms

ashutoshunfc

Improve